boothtaya.blogg.se - Roots of quadratic equation

Roots of quadratic equation pdf#
Roots of quadratic equation plus#

(ii) Putting x = 2, on the left side of the given equation, we get So, x = -1 is a root of the given equation. (i) Putting x = -1, on the left side of the given equation, we get

Roots of quadratic equation pdf#

We Are Providing You Free Pdf For 400+ Quadratic Equation Questions With Solution PDF Sets.

Determine whether these values are roots of the equations or not. If- 5 is a root of the quadratic equation 2x2 + px -15 0 and the quadratic equation p(x2 + x) + k 0 has equal roots, find the value of k. For Example, if ax + bx + c 0 then the root of the quadratic. x -b±(b2 4ac)/2a Example: The length of sides of a rectangle is given by x 3 and x 5 and the area of the rectangle is 3 unit2. The roots of the Quadratic equation is the value of an unknown factor of the equation. Finding the zeroes of the quadratic equations is known as solving the quadratic equation.Įxample : In each of the following quadratic equations the values of x are given. Method 1:The roots of the quadratic equations can be found by the Shridharacharaya formula. Every quadratic equation can have atmost two real roots.ģ). lets take a look at the graph of a quadratic function, and define a few new vocabulary words that are associated with. A quadratic function is always written as: f (x) ax2 + bx + c.

Since p(-2) = \((-2)^2\) + (-2) – 2 = 4 – 2 – 2 = 0.ġ). Since y mx + b is an equation of degree one, the quadratic function, y ax2 + bx + c represents the next level of algebraic complexity.

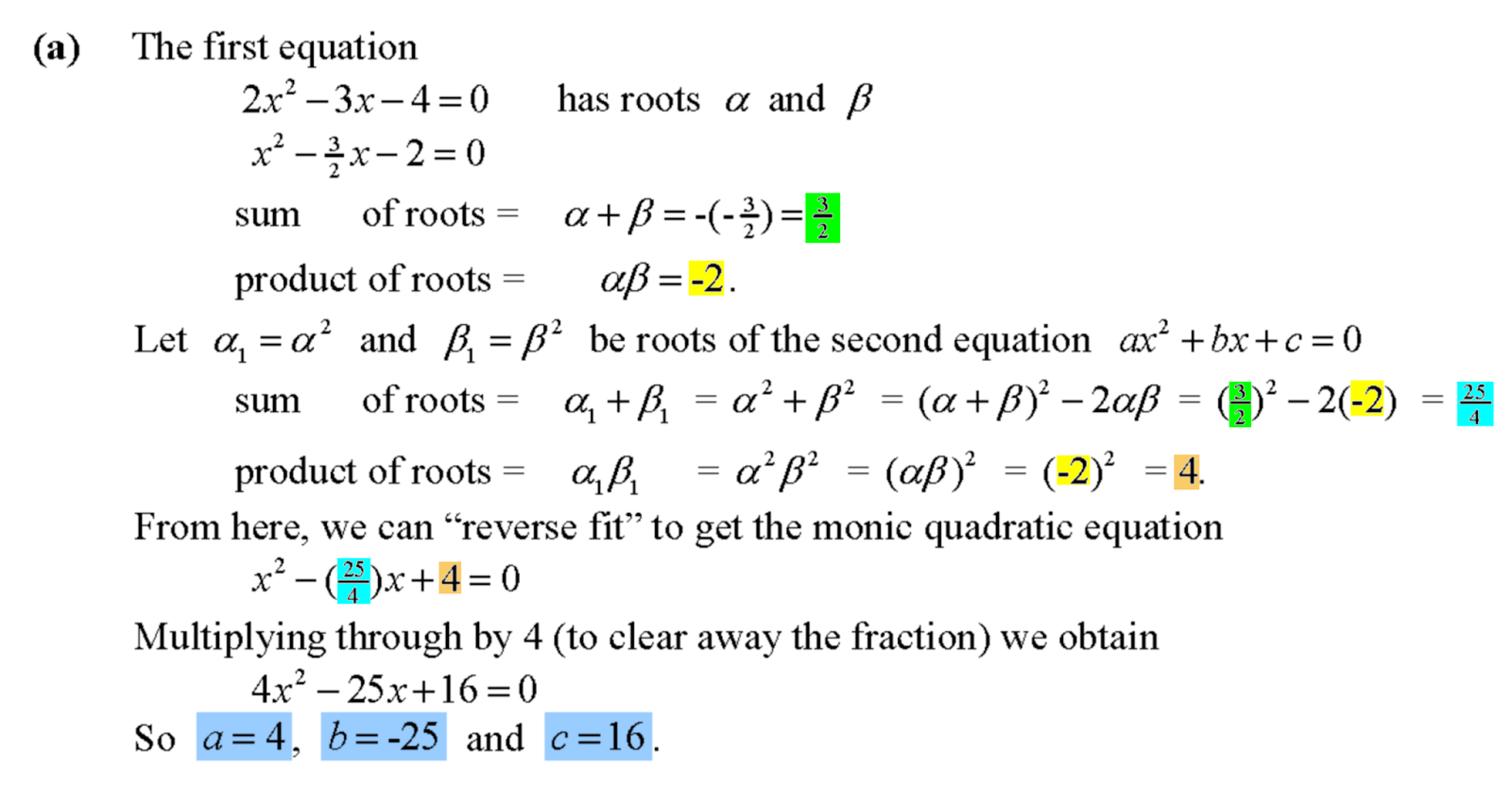
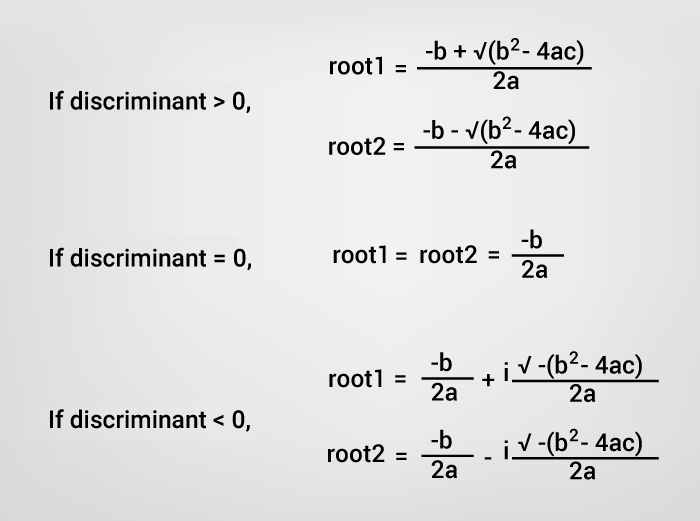
, is equal to the sum of the equations from the quadratic formula. These roots of the quadratic equation are also. From chapter one, we have also learnt that the sum of roots. If p(x) = 0 is a quadratic equation, then the zeroes of the poynomial p(x) are called the roots of the quadratic equation p(x) = 0. The roots of a quadratic equation are the two values of x, which are obtained by solving the quadratic equation. A quadratic equation will always have two roots. Let’s begin – Roots of Quadratic Equation A quadratic equation has two roots and the roots depend on the discriminant. Discriminant (D or Δ) or determinant just determines the nature of roots of a quadratic equation.Here you will learn what are the roots of quadratic equation with examples. The only relation which establishes between equal roots of two different quadratic equations are :ĭifference of two roots of a quadratic equation is : sqrt(D)/a which is not equal to D. X 2 - (Sum of two roots)x + (Product of two roots) = 0 So we can also write a quadratic equation in this form :Ī quadratic equation is written in this form : Let x and y be the two distinct roots of quadratic equation ax 2+bx+c = 0Īnd D = b 2-4ac then xy (Product of two roots)= c/a and x+y (Sum of two roots) = -b/a. How do we understand the nature of the roots of a quadratic equation Can we classify them Watch this video to know more To learn more about Quadratic Equ.

Roots of quadratic equation plus#

The first corresponds to the case when you have repeated roots (obviously) and the second occurs when a^2b^2 - 4a^3c - 1 = 0. When a, b, and c are real numbers, a 0 and the discriminant is zero, then the roots and of the quadratic equation ax2+ bx + c 0 are real and equal. And the quadratic formula tells us that if we have something in standard form like this, that the roots of it are going to be negative b plus or minus- so that. I'm not sure what your asking here, but the quadratic discriminant is \Delta = b^2 - 4ac.